KS1 & KS2 maths: place value

Make sure your class’s understanding of place value adds up with these activities from Mike Askew...

Sound understanding of place value is central to children’s understanding of the number system and the basis of sound mental and written calculation. It’s easy to think that once children have grasped the structure of hundreds, tens and units, it’s simply a case of applying this knowledge to larger numbers. But as children move up through primary school we also need to extend and deepen understanding.

Key Stage 1

A key understanding in KS1 is knowing how the position of a digit in a number gives its value. To adults this can seem almost ‘natural’, so it’s tempting to think that explaining then giving plenty of practice in saying or writing what digits ‘stand for’ will develop children’s understanding. However, I find many children learn the ‘trick’ of saying “There are six tens in 61” without proper understanding. A problem-solving context like ‘Market stalls’ helps children develop a deeper understanding.

Market stalls

I start off talking about market stalls and how one I go to sells fruit by the bowl. I tell the children that the stallholder told me about getting up early in the morning to go and buy boxes of fruit that she puts into bowls. She always puts 10 pieces of fruit into each bowl, and she might buy a crate of 63 bananas. How many bowls of 10 bananas will she be able to put out on her stall? And are there any left over?

I give pairs of children different quantities of fruit for them to work out how many bowls of 10 can be made, for example, 24, 37, 52 and so forth. As the problem is designed to introduce or extend children’s understanding of place value, I don’t expect them to know that 63 will allow for six bowls of 10 bananas straightaway.

As the children work at the problem, I list the number of pieces in the box, the number of bowls and the ‘leftovers’ on the board. Can the children see any patterns? This leads into a discussion of how our way of recording numbers allows us to easily know the number of groups of 10 in a number.

                                                                                     

Fruit	Bowls	Leftovers
24	2	4
37	3	7
52	5	2
61	6	1

Lower Key Stage 2

As children move from working with hundreds, tens and ones, the logical progression would seem to be to introduce thousands. The trouble with that is that some children come to think that as numbers get bigger the place value system is based on a grouping of four: thousands, hundreds, tens and ones. In fact it’s based on a grouping of three, which, somewhat paradoxically, is made easier to understand by working with really big numbers.

Big number boards

I make up three demonstration H, T, Os place value boards, taping these together in a zigzag fashion.

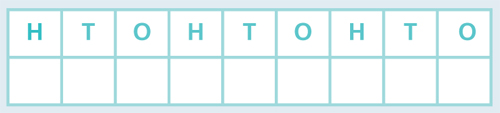
I write a nine-digit number on the board, deliberately making it difficult to read: e.g. 356728125

“Who can read this number?”

After we struggle to read the number I write it up on the place value board:

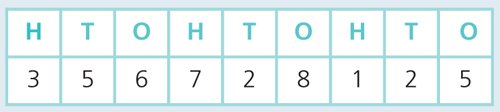
By folding the board at the joins, we can play with the power of reading the number in groups of three digits: three hundred and fiftysix million, seven hundred and twenty-eight thousand, one hundred and twenty-five. We talk about the repetition of the pattern of ‘hundreds and something’ millions, thousands or ones. We also talk about the values of the specific digits. The ‘7’ is ‘hundreds of thousands’ and the ‘3’ is ‘hundreds of millions’. The eight? ‘Ones of thousands’ seems a more ordinary way to talk than ‘units of thousands’, which is why I prefer hundreds, tens and ones to hundreds, tens and units.

Upper Key Stage 2

Some children think place value is only to do with whole numbers and that something different is going on with decimals. I prefer to help them see decimal numbers as an extension of what they’ve been doing as whole numbers get larger, only in reverse: things becoming 10 times smaller rather than bigger. Base Ten blocks can be used to model the relationships in multiplying or dividing by 10, 100 or 1000.

Powering up and down

Make a collection of Base Ten materials – small ones, ten-sticks, hundred ‘flats’ and thousand ‘blocks’ – and, literally, wrap them up. Newspaper is fine. By the time children reach Y5/6 they can find it difficult to think of, say, the 100 ‘flat’ as being anything other than 100. At the same time I don’t want the children to completely set aside what they know about the blocks, particularly in terms of the relationships between the different sizes.

Covering the blocks in paper makes them look sufficiently different for the children to think about them differently and yet be familiar enough to still draw on previous knowledge. I hold up a wrapped ten-stick, saying, “This is one.” Holding up a wrapped hundred square, I then ask, “So, if this is one (the stick), what value is this?”

The children usually know that the larger shape must be 10, and I don’t expect them to find this difficult. It’s the next question that we spend time discussing:

“What must happen to this ‘one’ (the stick) to become one ‘10’ (the flat)?”

Initial answers are usually that you need 10 of the smaller one to make one of the larger one. I make it clear that this is fine, but that it’s the answer to a different question: how many of the smaller ‘one’ are needed to make the larger ‘10’. I focus the children on imagining what must happen to the smaller ‘one’ to become the larger ‘10’.

In this way the conversation shifts from talking about the relationship between the two pieces as additive – you need 10 of those to make one of these – to multiplicative – the smaller piece needs to become 10 times larger.

Then we talk about the reverse relationship. What must happen to the ‘10’ to become the ‘one’? It would need to become 10 times smaller.

We play around with other relationships. What must happen to my ‘one’ to become the biggest piece (a hundred times larger), for the largest to become the ‘one’ (a hundred times smaller)?

Only after lots of this handling and talking about the blocks and the relationships between them do I record any of this. What I’m working on is that multiplying or dividing by 10, 100 or 1000 involves quantities becoming 10, 100 or 1000 times larger or smaller. A result of this growing or shrinking is that we position the same digits in different places to record these changes. But multiplying or dividing by 10, 100 or 1000 is not, in itself, about moving digits.

The scene is set for moving into decimals. If the ‘stick’ is ‘one’ what must happen to this to become the smallest piece? Ten times as small. We cannot simply write the digits for this to the right of the digits for the ‘ones’, hence the need for the decimal point.

Find out more

Mike Askew is Professor of Primary Education at Monash University, Melbourne and a freelance primary maths consultant. For fur ther information on his work, visit mikeaskew.net